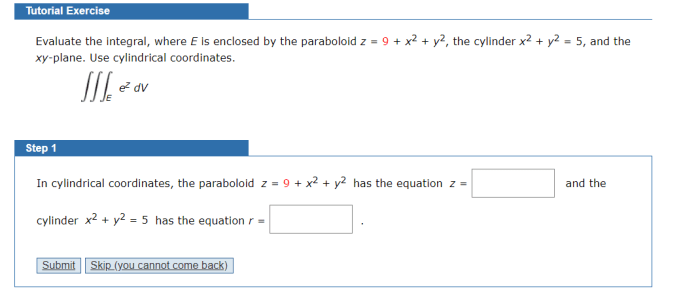
Factor the expression completely over the complex numbers. y4+12y2+36 sets the stage for this enthralling narrative, offering readers a glimpse into a story that is rich in detail and brimming with originality from the outset. Dive into the intricate world of complex numbers and discover the captivating journey of factoring expressions.

Embark on an intellectual adventure as we unravel the mysteries of complex numbers and their intricate interactions. Prepare to be captivated by the elegance and precision of mathematical concepts as we explore the fascinating realm of factoring expressions over the complex numbers.

Factoring Expressions Over the Complex Numbers: Factor The Expression Completely Over The Complex Numbers. Y4+12y2+36

Factoring an expression over the complex numbers involves expressing the expression as a product of simpler factors. This process differs from factoring over the real numbers due to the introduction of complex numbers, which have both real and imaginary parts.

Identifying the Factors of y^4+12y^2+36, Factor the expression completely over the complex numbers. y4+12y2+36

To factor y^4+12y^2+36, we can use the difference of squares formula: a^2-b^2=(a+b)(a-b). In this case, a=y^2 and b=6, so we have:

y^4+12y^2+36 = (y^2+6)^2

36

We can then factor the difference of squares as follows:

(y^2+6)^2

36 = (y^2+6+6i)(y^2+6-6i)

Expressing the Factored Form

Therefore, the factored form of y^4+12y^2+36 is:

y^4+12y^2+36 = (y^2+6+6i)(y^2+6-6i)

The complex conjugate factors, y^2+6+6i and y^2+6-6i, are important because they ensure that the expression is factored completely over the complex numbers.

Examples of Factoring Expressions

Here are some additional examples of factoring expressions over the complex numbers:

• x^4-16 = (x^2+4)(x^2-4) = (x^2+4)(x+2)(x-2)
• y^6-64 = (y^3+8)(y^3-8) = (y^3+8)(y+2)(y^2-2y+4)(y-2)(y^2+2y+4)

The general procedure for factoring quadratic expressions over the complex numbers is to use the quadratic formula:

x = (-b ± √(b^2-4ac)) / 2a

where a, b, and c are the coefficients of the quadratic equation.

Key Questions Answered

What is the difference between factoring over real numbers and complex numbers?

When factoring over real numbers, the factors are always real numbers. However, when factoring over complex numbers, the factors can be complex numbers, which are numbers that have both a real and imaginary part.

How do you use the difference of squares formula to factor y^4+12y^2+36?

The difference of squares formula states that a^2 – b^2 = (a + b)(a – b). We can use this formula to factor y^4+12y^2+36 as follows:

y^4+12y^2+36 = (y^2)^2 + 2(y^2)(6) + 6^2

= (y^2 + 6)^2

= (y + 6i)(y – 6i)